The constant Flux Linkage concept is of considerable importance in studying alternator transients. This concept is stated as – The Flux Linkage after a sudden disturbance in a closed circuit having zero resistance and zero capacitance remain constant at their predisturbed values.
There is no capacitance in the armature and the field windings of an alternator. Their resistances are negligibly small in comparison with their inductance. Thus, armature and field windings may be assumed to be purely inductive, and the flux linkages in the armature and field circuits cannot be changed immediately by the change of current in one winding must be accompanied by a change of current in the other to keep the flux linkages constant.
Proof of Constant Flux Linkage Theorem
The mesh voltage equations for any circuit can be written in the form given below:
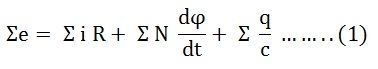 Using the symbol Ψ for the flux linkage (Nϕ), the equations may be written as follows:
Using the symbol Ψ for the flux linkage (Nϕ), the equations may be written as follows: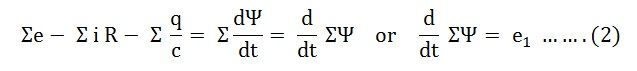 Where, e1 is the resultant voltage, which will be the function of the time.
Where, e1 is the resultant voltage, which will be the function of the time.
Integrating equation (2), the change in the linkage of flux from some arbitrarily chosen zero of time will be given by the equation shown below: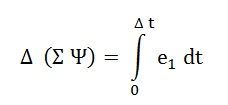 Where Δt is a small interval of time. As Δt tends to zero, so will be integral. Hence, ƩΨ = 0.
Where Δt is a small interval of time. As Δt tends to zero, so will be integral. Hence, ƩΨ = 0.
Therefore, the instantaneous change of flux linkage is zero.
